DictionaryChord |
| Buy sheetmusic at SheetMusicPlus |
A chord in music is any set of notes that is heard as if sounding simultaneously. These need not actually be played together: arpeggios and broken chords may for many practical and theoretical purposes be understood as chords. Chords and sequences of chords are frequently used in modern western, west African[1] and Oceanian[2] music, whereas they are absent from the music of many other parts of the world[3] The most frequently encountered chords in theory and music are triads, so called because they consist of three distinct notes: further notes may be added to give seventh chords, extended chords, or added tone chords. The most common chords are the major and minor triads and then the augmented and diminished triads. The descriptions "major", "minor", "augmented" and "diminished" are sometimes referred to collectively as chordal "quality". Chords are also commonly classed by their root note so, for instance, the chord C Major may be described as a three-note chord of major quality built upon the note C. However, since the structural meaning of a chord depends exclusively upon the degree of the scale upon which it is built,[4] chords are usually analysed by numbering them, using Roman numerals, upwards from the key-note. A chord may also be classified by its inversion, the order in which its notes are stacked from lowest to highest. There are four common ways of notating or representing chords[5] in western music other than conventional staff notation; Roman numerals, figured bass, much used in the Baroque era, macro symbols, sometimes used in modern musicology, and various systems of symbols and notations such as are typically found in the lead sheets, fake books and chord charts used in jazz and popular music to lay out the harmonic groundplan of a piece so that the musician may improvise, "jam", "vamp", "busk" or "head arrange" a part. 
C Major triad represented in staff notation.
Definition and historyMain article: Harmony
The English word "chord" derives from "cord", a Middle English shortening of "accord" in the sense of "in tune with one another". For a sound configuration to be recognized as a chord it must have a certain duration. Since a chord may be understood as such even when all its notes are not simultaneously audible there has been some academic discussion regarding the point at which a group of notes can be called a chord. Jean-Jacques Nattiez (1990, p. 218) explains that "we can encounter 'pure chords' in a musical work," such as in the "Promenade" of Modest Mussorgsky's Pictures at an Exhibition but "often, we must go from a textual given to a more abstract representation of the chords being used" - as in Claude Debussy's Première Arabesque. 
Upper stave: Claude Debussy's Première Arabesque. The chords on the lower stave are constructed from the notes in the actual piece, shown on the upper stave.
Early Christian harmony featured the perfect intervals of a fourth, a fifth, and an octave. In the 15th and 16th centuries, the major and minor triads became increasingly common, and were soon established as the default sonority for Western music. Such triads can be described as a series of three notes; the root note, the "third", and the "fifth" of the chord. As an example, the C major scale consists of the notes C D E F G A B C while the chord of C Major - the major triad formed using the note C as the root - consists of C itself (the root note of the scale), E (the third note of the scale) and G (the fifth note of the scale). This triad is major because the interval from C to E, of four semitones, is a major third. Using the same scale a chord may be constructed using the D as the root note; D (root), F (third), A (fifth). While there were four semitones between the root and third of the chord on C, in the D chord there are only 3 semitones between the root and third (the outer notes are still a perfect fifth apart). Thus, while the C triad is major, the D triad is minor. A triad can be constructed on any note of the C major scale and all will be minor or major, with the exception of the triad on the leading-tone which is diminished. Taking any other major scale (Ionian mode), the first, fourth and fifth intervals, when used as roots, form major triads. Similarly, as any major scale can also yield a relative minor, in any natural minor scale (Aeolian mode) minor triads are found on the tonic, fourth and fifth degrees of the scale. Each seven-note diatonic scale can provide three major and three minor chords, both sets of three standing in the same I-IV-V relationship to one another. The seventh degree of the major (degree two of the relative minor) will result in a diminished chord. See Music and mathematics#Mathematics of musical scales. Four-note "seventh chords" were widely adopted from the 17th century. The harmony of many contemporary popular Western genres continues to be founded in the use of triads and seventh chords, though far from universally. Notable exceptions include chromatic, atonal or post-tonal contemporary classical music (including the music of some film scores) and modern jazz (especially circa 1960), in which chords often include at least five notes, with seven (and occasionally more) being quite common. Polychords are formed by two or more chords superimposed. Often these may be analysed as extended chords but some examples lack the tertian sonority of triads (See: altered chord, secundal chord, quartal and quintal harmony and Tristan chord). A nonchord tone is a dissonant or unstable tone that lies outside the chord currently heard, though often resolving to a chord tone. A succession of chords is called a chord progression. Chord characteristicsEvery chord has certain characteristics, which include:
Number of distinct notesChords may be classified according to the number of notes they contain. More precisely, since instances of any given note in different octaves may be taken as the same note for the purposes of analysis, it is better to speak of the number of distinct pitch classes used in their construction. Three such pitch classes are needed to define any common chord, therefore the simultaneous sounding of two notes is sometimes classed as an interval rather than a chord. Hence Andrew Surmani (2004, p. 72) states; "when three or more notes are sounded together, the combination is called a chord" and George T. Jones (1994, p. 43) explains; "two tones sounding together are usually termed an interval, while three or mores tones are called a chord" while, according to Monath (1984, p. 37); "A chord is a combination of three or more tones sounded simultaneously for which the distances (called intervals) between the tones are based on a particular formula." Chords, however, are so well-established in Western music that sonorities of two pitches, or even single-note melodies, are commonly heard as "implying" chords, a psychoacoustic phenomenon resulting from a lifetime of exposure to the conventional harmonies of music so that the brain "completes" the chord.[6] Otto Karolyi[7] writes that "two or more notes sounded simultaneously are known as a chord." Two-note combinations, whether referred to as chords or intervals, are called dyads. Chords constructed of three notes of some underlying scale are described as triads. They may be understood to be constructed from a stack of two third intervals. Chords of four notes are known as tetrads, those containing five are called pentads and those using six are hexads. Sometimes the terms "trichord", "tetrachord", "pentachord" and "hexachord" are used, though these more usually refer to the pitch classes of any scale, not generally played simultaneously. Chords that may contain more than three notes include suspended chords, pedal point chords, dominant seventh chords and others termed extended chords, added tone chords, clusters, and polychords. Scale degreeIn the key of C major the first degree of the scale, called the tonic, is the note C itself, so a C major chord, a triad built on the note C, may be called the one chord of that key and notated in Roman numerals as I. The same C major chord can be found in other scales: it forms chord III in the key of A minor (A-B-C) and chord IV in the key of G major (G-A-B-C). This numbering lets us see the job a chord is doing in the current key and tonality. Many analysts use lower-case Roman numerals to indicate minor triads and upper-case for major ones, and "degree" and "plus" signs ( o and + ) to indicate diminished and augmented triads respectively. Otherwise all the numerals may be upper-case and the qualities of the chords inferred from the scale degree. Chords outside the scale can be indicated by placing a flat/sharp sign before the chord — for example, the chord of E flat major in the key of C major is represented by ♭III. The tonic of the scale may be indicated to the left (e.g. F♯:)or may be understood from a key signature or other contextual clues. Indications of inversions or added tones may be omitted if they are not relevant to the analysis. Roman numerals indicate the root of the chord as a scale degree within a particular major key as follows:
When a chord is analysed as "borrowed" from another key it may be shown by the Roman numeral corresponding with that key after a slash so, for example, V/V indicates the dominant chord of the dominant key of the present home-key. The dominant key of C major is G major so this secondary dominant will be the chord of the fifth degree of the G major scale, which is D major. If used, this chord will cause a modulation. InversionMain article: Inversion (music)
In the harmony of Western art music a chord is said to be in root position when the tonic note is the lowest in the chord, and the other notes are above it. When the lowest note is not the tonic, the chord is said to be inverted. Chords, having many constituent notes, can have many different inverted positions as shown below for the C major chord:
Further, a four-note chord can be inverted to four different positions by the same method as triadic inversion. Where guitar chords are concerned the term "inversion" is used slightly differently; to refer to stock fingering "shapes".[8] Secundal, tertian, and quartal chordsMany chords are a sequence of ascending notes separated by intervals of roughly the same size. For example the C major triad's notes, C-E-G, are defined by a sequence of two intervals, the first (C-E) being a major third and the second (E-G) a minor third. Any such chord that can be decomposed into a series of (major or minor) thirds is called a tertian chord. Most common chords are tertian. A chord such as C-D-E♭, though, is a series of seconds, containing a major second (C-D) and a minor second (D-E♭). Any such chord that can be decomposed into a series of (major or minor) seconds is called tertian. The chord C-F-B, consists of a perfect fourth C-F and an augmented fourth (tritone) F-B. Any such chord that can be decomposed into a series of (perfect or augmented) fourths is called quartal. These terms can become ambiguous when dealing with non-diatonic scales such as the pentatonic or chromatic scales. The use of accidentals can also complicate the terminology. For example the chord B♯-E-A♭ appears to be a series of diminished fourths (B♯-E and E-A♭) but is enharmonically equivalent to (and sonically indistinguishable from) the chord C-E-G♯, which is a series of major thirds (C-E and E-G♯). See also Mixed-interval chord. Triads
Pitch constellations of triads
Main article: Triad (music)
Triads, also called triadic chords, are tertian chords (see above) with three notes. The four basic triads are described below.
Seventh chords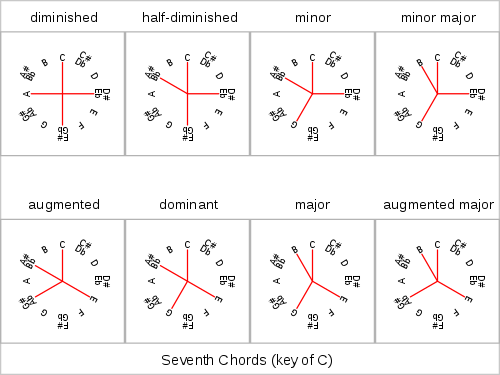
Pitch constellations of seventh chords.
Main article: Seventh chord
Seventh chords are tertian chords (see above), constructed by adding a fourth note to a triad, at the interval of a third above the fifth of the chord. This creates the interval of a seventh above the root of the chord, the next natural step in composing tertian chords. The seventh chord on the fifth step of the scale (the dominant seventh) is the only one available in the major scale: it contains all three notes of the diminished triad of the seventh and is frequently used as a stronger substitute for it. There are various types of seventh chords depending on the quality of both the chord and the seventh added. In chord notation the chord type is sometimes superscripted and sometimes not (e.g. Dm7, Dm7, and Dm7 are all identical).
Extended chordsMain article: Extended chord
Extended chords are triads with further tertian notes added beyond the seventh; the ninth, eleventh, and thirteenth chords. After the thirteenth any notes added in thirds will duplicate notes elsewhere in the chord: all seven notes of the scale are present in the chord and further added notes will not give new pitch classes. Such chords may be constructed only by using notes that lie outside the diatonic seven-note scale (See #Altered chords below).
Other extended chords follow the same rules as shown above, so that for example Maj9, Maj11 and Maj13, shown above are with major sevenths rather than minor sevenths: similarly m9, m11 and m13 will have minor thirds and minor sevenths. Altered chordsMain article: Altered chord
Although the third and seventh of the chord are always determined by the symbols shown above, the fifth, ninth, eleventh and thirteenth may all be chromatically altered by accidentals (the root cannot be so altered without changing the name of the chord, while the third cannot be altered without altering the chord's quality). These are noted alongside the element to be altered. Accidentals are most often used in conjunction with dominant seventh chords. "Altered" dominant seventh chords (C7alt) may have a flat ninth, a sharp ninth, a diminished fifth or an augmented fifth (see Levine's Jazz Theory). Some write this as C7+9, which assumes also the flat ninth, diminished fifth and augmented fifth (see Aebersold's Scale Syllabus). The augmented ninth is often referred to in blues and jazz as a blue note, being enharmonically equivalent to the flat third or tenth. When superscripted numerals are used the different numbers may be listed horizontally (as shown) or else vertically.
Added tone chordsMain article: Added tone chord
An added tone chord is a triad chord with an added, non-tertian note, such as the commonly added sixth as well as chords with an added second (ninth) or fourth (eleventh) or a combination of the three. These chords do not include "intervening" thirds as in an extended chord. Added chords can also have variations. Thus madd9, m4 and m6 are minor triads with extended notes. Added-sixth chords can be considered as belonging to either of two separate groups; chords that contain the sixth (from the root) as a chord member—a note separated by the interval of a sixth from the chord's root—and inverted chords in which the interval of a sixth appears above a bass note that is not the root[citation needed]. The major sixth chord (also called, sixth or added sixth with the chord notation 6, e.g., "C6") is by far the most common type of sixth chord of the first group. It comprises a major triad with the added major sixth above the root, common in popular music[5]. For example, the chord C6 contains the notes C-E-G-A. The minor sixth chord (min6 or m6, e.g., "Cm6") is a minor triad with the same added note. For example, the chord Cmin6 contains the notes C-E♭-G-A. In chord notation, the sixth of either chord is always assumed to be a major sixth rather than a minor sixth, however a minor sixth interval may be indicated in the notation as, for example, "Cm(m6)", or Cmm6. The augmented sixth chord usually appears in chord notation as its enharmonic equivalent, the seventh chord. This chord contains two notes separated by the interval of an augmented sixth (or, by inversion, a diminished third, though this inversion is rare). The augmented sixth is generally used as a dissonant interval most commonly used in motion towards a dominant chord in root position (with the root doubled to create the octave to which the augmented sixth chord resolves) or to a tonic chord in second inversion (a tonic triad with the fifth doubled for the same purpose). In this case, the tonic note of the key is included in the chord, sometimes along with an optional fourth note, to create one of the following (illustrated here in the key of C major):
The augmented sixth family of chords exhibits certain peculiarities. Since they are not based on triads, as are seventh chords and other sixth chords, they are not generally regarded as having roots (nor, therefore, inversions), although one re-voicing of the notes is common (with the namesake interval inverted to create a diminished third). The second group of sixth chords includes inverted major and minor chords, which may be called sixth chords in that the six-three (6/3) and six-four (6/4) chords contain intervals of a sixth with the bass note, though this is not the root. Nowadays this is mostly for academic study or analysis (see figured bass) but the neapolitan sixth chord is an important example; a major triad with a flat supertonic scale degree as its root that is called a "sixth" because it is almost always found in first inversion. Though a technically accurate Roman numeral analysis would be ♭II, it is generally labelled N6. In C major, the chord is notated (from root position) D♭, F, A♭. Because it uses chromatically altered tones this chord is often grouped with the borrowed chords (see below) but the chord is not borrowed from the relative major or minor and it may appear in both major and minor keys.
Suspended chordsMain article: Suspended chord
A suspended chord, or "sus chord" (sometimes wrongly thought to mean sustained chord), is a chord in which the third is delayed by either of its dissonant neighbouring notes, forming intervals of a major second or (more commonly) a perfect fourth with the root. This results in two distinct chord types: the suspended second (sus2) and the suspended fourth (sus4). The chords, Csus2 and Csus4, for example, consist of the notes C D G and C F G, respectively. The name suspended derives from an early polyphonic technique developed during the common practice period, in which a stepwise melodic progress to a harmonically stable note in any particular part was often momentarily delayed or suspended by extending the duration of the previous note. The resulting unexpected dissonance could then be all the more satisfyingly resolved by the eventual appearance of the displaced note. In traditional music theory the inclusion of the third in either chord would negate the suspension, so such chords would be called added ninth and added eleventh chords instead. In modern layman usage the term is restricted to the displacement of the third only and the dissonant second or fourth no longer needs to be held over ("prepared") from the previous chord. Neither is it now obligatory for the displaced note to make an appearance at all though in the majority of cases the conventional stepwise resolution to the third is still observed. In post-bop and modal jazz compositions and improvisations suspended seventh chords are often used in nontraditional ways: these often do not function as V chords, and do not resolve from the fourth to the third. The lack of resolution gives the chord an ambiguous, static quality. Indeed, the third is often played on top of a sus4 chord. A good example is the jazz standard, "Maiden Voyage". Extended versions are also possible, such as the seventh suspended fourth which, with root C, contains the notes C F G B♭ and is notated as C7sus4 Notation
C Major triad represented in staff notation.
Chords can be represented in many and various different ways. The most common notation systems are:[5]
Roman numeralsMain article: Roman numerals#Music theory
While scale degrees are typically represented with Arabic numerals, often modified with a caret or circumflex, the triads that have these degrees as their roots are often identified by Roman numerals (see also diatonic functions). Since the 1970s, upper-case Roman numerals indicate major triads while lower-case Roman numerals indicate minor triads, as the following chart illustrates. Some writers, (e.g. Schoenberg) however, use upper case Roman numerals for both major and minor triads. Lower-case Roman numerals with a degree symbol indicate diminished triads. For example, in the major mode the triad on the seventh scale degree, the leading tone triad is diminished. Some writers use upper-case Roman numerals to indicate the chord is diatonic in the major scale, and lower-case Roman numerals to indicate that the chord is diatonic in the minor scale.
Also:
In performance practice, individual strings of stringed instruments, such as the violin, are often denoted by Roman numerals, with higher numbers denoting lower strings. For example I signifies the E string on the violin and the A string on the viola and cello, these being the highest strings, respectively, on each instrument. They are also sometimes used to signify position. In this case, the number in Roman numerals corresponds with the position number. For example, III means third position and V means fifth. Figured bass notationMain article: Figured bass
Figured bass, or thoroughbass, is a kind of integer musical notation used to indicate intervals, chords, and nonchord tones, in relation to a bass note. Figured bass is closely associated with basso continuo, an accompaniment used in almost all genres of music in the Baroque period, though rarely in modern music. A part notated with figured bass consists of a bass-line notated with notes on a musical staff plus added numbers and accidentals beneath the staff to indicate at what intervals above the bass notes should be played, and therefore which inversions of which chords are to be played. The numbers indicate the number of scale steps above the given bass-line that a note should be played. For example: Here, the bass note is a C, and the numbers 4 and 6 indicate that notes a fourth and a sixth above it should be played, that is an F and an A. In other words, the second inversion of an F major chord is to be played. In cases where the numbers 3 or 5 would normally be indicated, these are usually (though not always) left out, owing to the frequency these intervals occur. For example: In this sequence, the first note has no numbers accompanying it—both the 3 and the 5 have been omitted. This means that notes a third above and a fifth above should be played—in other words, a root position chord. The next note has a 6, indicating a note a sixth above it should be played; the 3 has been omitted—in other words, this chord is in first inversion. The third note has only a 7 accompanying it; here, as in the first note, both the 3 and the 5 have been omitted—the seven indicates the chord is a seventh chord. The whole sequence is equivalent to: For further details, see the main article. Macro analysisMain article: Macro analysis
In macro analysis, uppercase or lowercase letters are used to indicate the roots of chords, followed by symbols which specify the chord quality.[5]
Jazz and pop notationMain article: Chord names and symbols (jazz and pop music)
In jazz and pop notation, a chord name and the corresponding symbol are typically composed of one or more of the following parts:
For instance, the name C augmented seventh, and the corresponding symbol Caug7, or C+7, are both composed of parts 1, 2, and 3. Chord quality
Major, minor, augmented, and diminished chords
Rules to decode chord names and symbols
Examples
Borrowed chordsMain article: Borrowed chord
A borrowed chord is one that is taken from a different key to that of the piece it is used in (called "home key"). The most common occurrence of this is where a chord from the parallel major or minor key is used. Particularly good examples can be found throughout the works of composers such as Schubert. For instance, for a composer working in the C major key, a major ♭III chord would be borrowed, as this appears only in the C minor key. Although borrowed chords could theoretically include chords taken from any key other than the home key, this is not how the term is used when a chord is described in formal musical analysis. Progression
Main article: Chord progression
Whenever different chords are played in sequence they can be described as a chord progression (or harmonic progression). Chord progressions are frequently used in Western music.[3] A chord progression "aims for a definite goal" of establishing (or contradicting) a tonality founded on a key, root or tonic chord.[4] The study of harmony involves chords and chord progressions, and the principles of connection that govern them.[11] See also
References
Further reading
External links
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
This article is licensed under the GNU Free Documentation License. It uses material from the Wikipedia article "Chord". Allthough most Wikipedia articles provide accurate information accuracy can not be guaranteed. | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Help us with donations or by making music available!
©2023 Classic Cat - the classical music directory

Debussy, C.
2 arabesques
Chris Breemer
Beethoven, L. van
Octet for Oboes, Clarinets, Horns & Bassoons
Soni Ventorum Wind Quintet
Tchaikovsky, P.I.
Violin Concerto
Nathan Milstein
Mendelssohn-Bartholdy, F.
6 Songs op. 34
Valentina Valente
Mozart, W.A.
Mass "Great Mass" in C minor
Singakademie Tsukuba
Tchaikovsky, P.I.
Swan Lake (suite)
Harmonie des Deux Rives





 as G is a 5th above C and E is a 3rd above C
as G is a 5th above C and E is a 3rd above C as C is a 6th above E and G is a 3rd above E
as C is a 6th above E and G is a 3rd above E as E is a 6th above G and C is a 4th above G
as E is a 6th above G and C is a 4th above G


